Development of place value - concepts, sample activities, assessment, and evaluation

Place value concepts develop along with other number sense concepts (prenumber sense (subitizing, counting, conservation ... ) and number sense (cardinality, operations ... )). All, are intwined as they develop over months and years first with smaller whole numbers and later with larger whole numbers and other numbers.

Counting past ten, is a student's first encounter of place value, however, they don't recognize it as place value, just another number in the continuation of counting. Skip counting by tens is memorized, by young children, and is followed by knowing ten is a group ten objects. However, unitizing, takes a few more years to conceptualize. So students count first to ten, then twenty, and later to 100. To develop a good number sense, of the difference between 1 - 10 and 20 - 100 takes additional time. Likewise, 100 - 1000, takes even more time to understand the number of ones and tens between 100 and 1,000 is many more than between 1 and 100. Later they will discover the pattern for counting whole numbers and be able to use it to count or represent large finite objects, and even later understand counting can be, theoretically infinite. Next, they will discover these ideas can extend to the infinitesimal with decimal numbers, rational numbers, powers of ten, and other numbers. If, they continue their study of place value and powers they can understand different numeral systems and bases.

This page reviews developmental landmarks related to place value.

Ten is a big number

No place value understanding. Children see ten as the same kind of number as 1,2,3, ... 9, only bigger. Can count to ten and say they have ten fingers or ten toes. Most likely do not conserve or have cardinality.

• Ten is a bigger number than 1, 2, 3, ... 9.
• Ten is less than 11, 12, ....

Grouping by ten

Children can use one-to-one relationships and systematically count objects accurately to at least twenty. They can also skip count by tens and understand skip counting as repeated addition. Not unitize multiples of ten. There is no place value understanding. Students understand numbers can be grouped with different combinations and ten is just one of several groupings (3 + 2; 5 + 2; ... ). Students are easily confused by the one in teens and two in twenties as one and two rather than ten or twenty.  

When asked they will represent numbers greater than ten with a one-to-one relationship For example: showing how many students in their class by drawing designs so one design represents one student. Not groups of tens and ones. They are working toward unitizing (counting equal groups of objects with a one-to-one strategy; like counting five groups of ten by 1, 2, 3, 4, 5 and recognizing it as five equal groups of ten or 50) but have not yet achieved it.

• Objects can be grouped in tens.
• Grouping by tens, makes it easier to count.
• Objects can be grouped into tens and leftovers.

Place value models should use actual objects and put them into groups of ten.

Students can inventory books in the classroom by creating stacks of ten books and can recognize the groups of ten as units as well as a group of ten, but not simultaneously. Some may even say, they know it is ten and ten ones, but will be serious that they are equal, but not the same thing. Therefore, can total books as groups of ten and extras.

Pre place value

Students understand number values for whole numbers less than 20, cardinality to 100, can order and sequence numbers to 100, know zero is the absence of objects, understand hierarchical inclusion of numbers in a counting sequence, and more than one addition fact relates to a number's value (but not hierarchical inclusion of addition related to number sense), and those facts can be used to compose and decompose numbers.

• Ten is a group of ten ones.
• Objects can be put into groups of ten and counted by skip counting by tens.
• Counting by tens is helpful.
• Counting by tens is like counting by ones with zeros.
• Objects can be grouped into tens when thinking of number value and to solve problems. (significance of ten)
• Numbers can be grouped as ten and more.

Students group objects by tens and consider it as an additive process, the same as putting objects into other groupings (pairs, fives, eight, four...). Not as a significant step to creating a number system with palce value.

Solves addition and subtraction problems mentally by decomposing into groups of tens and ones, composing the tens, then the ones, and adding the tens and ones.

May or may not know how zero is used to mark positions with no value.

Students continue to act on groups of ten and a hundred as if they are two separate things and can develop skill in doing it with small numbers of tens and maybe one hundred.

Values with several multiples of ten (30-90) and hundreds (200-900) are though of as large groups, but not with an accurate model.

Therefore, it is important they see and manipulate place value models that are proportional. Ten strips, centimeter models: 1 centimeter cubes, 10 centimeter rods, and 100 centimeter squares, and later 1000 centimeter cubes. It is very important learners have many experiences with these so they can easily visualize values more accurately.

Unitize

In the middle elementary grades learners can begin to unitize. To do so, they must simultaneously understand a group represents two different values. In place value this means they understand each place can simultaneously have two values: tens and ten ones; hundred and hundred ones or ten tens; one tenth or one tenth of one or ten hundredths.

Unitizing is also necessary when working with measurement:

• Money, nickel is equal to 5 pennies;
• Linear measurement, 100 centimeters is equeal to 1 meter; 12 inches is equal to 1 foot;
• Area, one square foot is equal to 144 suare inches
• Volume, one cubic yard is equal to 27 cubic feet
• Time one hour is equal to 60 minutes;
• Other measurements, 12 eggs is equal to 1 dozen.

However, the topic here is place value so here are some more place value examples:

• Understand groups of ten are simultaneously both: equal groups of ten and the equivalent number of units or ones.
  • Ten represents one group of ten;
  • (* * * * * * * * * *) and 
  • Ten units
  • * * * * * * * * * *
  • 10
• Twenty; 20. The two has the value of 2 tens and 20 units.
  • Two represents two groups of ten;
  • (* * * * * * * * * *) (* * * * * * * * * *) and 
  • Twenty units
  • * * * * * * * * * * * * * * * * * * * *
  • 20. 
• In 32 the three means three groups of ten or thirty units plus two additional units (ones) represented by the two.
  • Three represents three groups of ten;
  • (* * * * * * * * * *) (* * * * * * * * * *) (* * * * * * * * * *) and 
  • Thirty units
  • * * * * * * * * * * * * * * * * * * * * * * * * * * * * * * and
  • Two units. 
  • * *
  • Thirty + 2; 32 units
• In 346; the three means three groups of one-hundred or three-hundred units, plus four groups of ten or forty units, plus six additional units (ones), 300 + 40 + 6; 346
  • Three represents three groups of one-hundred;
  • (* 100 *) (* 100 *) (* 100 *) and 
  • Three hundred units
  • (* 300 *)
  • Four represents four groups of ten. 
  • (* 10 *) (* 10 *) (* 10 *) (* 10 *)
  • Forty units
  • (* 40 *)
  • Six units
  • (* * * * * *)
  • Three hundred + forty + 6; 346
  • 300 + 40 + 6; 346

Place value relationships

For students to completely understand place value, they must unitize. An activity to assess young students understanding of place value is:

Provide a child a group of objects to count (beans, cubes, tokens). Select a number between 23 - 46. Ask the learner to count out a number of objects: 24. When they finish, ask them to write the total (24) on a piece of paper. If they wrote 24, then tell them, that's 24. Good. Now I want you to use the 24 counters and show me what the two (point to the two) and the four (point to the four) represent (in 24) with the counters. If they don't respond follow up with. Show me what objects go with the 2 and what objects go with the 4.

I have observed hundreds of young children interviewed with this activity and most observations will either fall into one of two categories.

1. When given 24 objects and asked to show what the 2 and 4 represent in 24, they will put two counters by the 2 and four counter by the 4. When asked what about the other 18, they will either slide them aside or look befuddled. 
2. Others will easily unitize, know, and explain the 2 is 2 tens while saying it can also be, at the same time (simultaneously), 20 tiles, or 20 units/ ones, and the four represents or stands for 4 tiles. Together 24 tiles. 

Young learners who understand place value will have a good sense of number values. They easily round numbers to multiples of ten and can count forward and backward by tens and hundreds starting with any number. They know multiples of ten include 10 X 10, and maybe 10 X 100.

They also know:

• Place value unitizes by tens, hundreds, thousands, ...
• Usually have a real good working understanding of number values to at least 1000.
• Numbers can be located to a nearest number with a value of ten (rounding).
• Ten has a significant role in our base ten number system. Ten, hundred, and thousand are important multiples of this number system.
• A number system is a code that uses a set of symbols (numerals) and rules for combining the symbols to represent number values.
• All numbers can be represented with only ten symbols or numerals.
• Ten numbers, represent multiple units of ten (tens, hundreds, …).
• Zero has an important function in writing numbers.
• Position of a digit can represent different values.
• The sum of the value of all digits determines the value of a numeral (cardinality) The total value of a numeral is the sum of all digits values in its particular place. A digits value is a function of its number value and it's place value.
• Numbers can be composed and decomposed into different equivalent groups of tens and more (taken apart (decomposed) and put together in different order (composed) or regrouped and written in expanded notation.
  • Understand groups can be regrouped as other equivalent groups. Five groups of ten is also two groups of ten, and three groups of ten.
  • They are able to switch from one to the other, easily recognizing the equality of the values and the interchangeability of factors as single units or equal groups of those units. 
• Numbers of groups can be operated on without regard to the value of the group (62 - 41), (60 - 40) can be operated as (6 - 4) and (2 - 1) and write, think, or say 21 without really (multiplying by ten). This thinking about and working with groups is a result of unitizing.
  • Can count each group as one unit (1 of 10, 2 of 10, 3 of 10 ...) and realize each unit has and must have an equivalent number of objects in it.
  • Know that five groups of ten is fifty. They don't need to count by tens five times to know, five groups is 50.
  • They know the two in 24 is simultaneously 2 tens and twenty. They no longer have have a problem, as young children do, understanding the place value of the two and four, in 24 (2 tens and 4 ones). 
• Addition facts can often use ten as an anchor (32; 30 +2) for addition and subtraction. This is helpful when adding (27 + 35); (27 + 30) + 5; (57 + 3) + 2; 62 both for adding or subtracting to make tens, and adding and subtracting in leaps of ten.
• All place values are multiples of 10 (10, 100, 1000, ...)

As learners have numerous experiences with proportional models to learn place value of whole numbers the same models can be used to develop visual models for decimals and place value.

Place value as decimals and exponential values

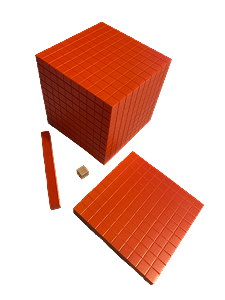
Ideas to support students learn place value decimals less than one and exponents.

Have students skip count different decimal multiples:

• .1, .1, .2, .3, .4 ...
• .01, .01, .02, .03, .04, ...
• .001, .001, .002, .003, .004, ...

Assess Unitizing of place values with expanded notation and asking the value of each digit in decimal numbers.

• In the number .1. What place is the one in and what is its value? tenths and one tenth
• In the number .21. What place is the two in and what is its value? tenths and two tenths
• What is the value of 1 digit in .1? one tenth
• What is the value of each digit in .2? two tenths
• What is the value of each digit in .24? two hundredths and four hundredths
• What is the value of each digit in .12? 1 tenth and 2 hundredths
• What is the value of each digit in .123? .1 + .02 + .003

Use mental addition problems like:

• Start at .1 what is .2 more? .3
• Start at .01 what is .02 more? .03
• Start at .01 what is .1 more? .11
• Start at .05 what is .001 more? .051

Use money

• $.20 Is how many dimes? two dimes
• $.20 Is how many pennies? 20 pennies
• 2 dimes is how much of a dollar? .2 of a dollar
• .2 of a dollar is how many dimes? 2 dimes
• 3 pennies is how much of a dime? .3 of a dime
• 3 pennies is how much of a dollar? .03 of a dollar

Connect multiplication of decimals to fractions. Repeat these problems with visual models until students automatically connect multiplicaiton of a whole number by tenths is tenths. Then repeat with the same kinds of problems for whole numbers and hundredths. 2 x 1/100 and 1/100 of 1 and ... Then 1/10 of 1/10; 1/100; and 2/10 of 2/10; 4/100 ... Then tenths of hundredths ...

• 2 x 1/10; is .2
• 1/10 of 1; is .1
• 1/10 of 2; is .2

Do the same kinds of problems with graph paper that is 10x10 or 10x100, 100x100 if want to get to 10,000ths ...

Can also use Cuisenaire rods, squares, and cubes. Use each of the following, in turn, (white cube, orange rod, orange square, and orange cube) to represent one unit and have the students determine what the others. Have them let one vary for each and tell what different collections are. E.g. if an Orange cube is one what is an orange square? an orange rod, A white cube?, Then ask what .1, .01, or .001 of each would be and what if multiply?

Also have had them use calculators to check and see if the calculator agrees with their reasoning.

I have found that students who know number values and can read and write numerals well (see reading, writing, and saying numbers lesson will know what the answer is and place the decimal point without counting (for decimal numbers in tenths, hundredths, and thousandths). And if they know that, then they can always figure the rule or procedure for larger numbers, if they need to.

Us proportional models: (Cuisenaire models pictured)

• 1 cm x 1 cm x 1cm cube (white)
• 1 cm x 1 cm x 10 cm rod (orange)
• 1 cm x 10 cm x 10 cm square (orange)
• 10 cm x 10 cm x 10 cm cube (orange)

See also Place value number line lesson plan

• Place value is exponential: 10⁰,10¹,10², 10³...
  (50 = 5 * 10¹+0*10⁰
  51 = 5 * 10¹+1*10⁰,
  52 = 5 * 10¹+2*10^0,
  200= 2*10² +0*10¹ + 0*10⁰,
  201=2*10²+0*10¹ + 1*10⁰,
  234=2*10²+ 3*10¹+4*10⁰)
• Decimals as exponential: 10^-1,10^-2, 10^-3
• Unitizing place values of decimal numbers, less than and greater than one, as an exponential progressions.
• Hindus are credited with the invention of a place value system.
• The Arabs are credited with applying and spreading its use.
• Translations of Fibonacci's work introduced it to Europe.

Worksheets

White cube with a rod, square, and cube

Materials:

• 1 cm x 1 cm x 1cm cube (white)
• 1 cm x 1 cm x 10 cm rod
• 1 cm x 10 cm x 10 cm square
• 10 cm x 10 cm x 10 cm cube (orange)

Use the four centimeter blocks to answer the following questions:
Let one white cube = 1

If a white cube = 1, then
one orange rod =

If a white cube = 1, then
one orange square =

If a white cube = 1, then
one orange cube =

If one white cube = 1, then what is the value of each of the following sets?

1 orange square, 2 orange rods, and 4 white cubes

8 orange squares and 3 white cubes

2 orange rods, and 8 white cubes

5 orange cube, 2 orange rods, and 4 white cubes

7 white cubes

1 orange square, 3 orange rods, and 2 white cubes

5 orange cubes, 4 orange squares, 6 white rods, and 3 white cubes

How many cubes, squares, and rods are needed to represent the following numerals?

.1

.3

.4

.7

1.4

1.5

2.5

12.6

Rod with a white cube, square, and cube

Materials:

• 1 cm x 1 cm x 1cm cube (white)
• 1 cm x 1 cm x 10 cm rod
• 1 cm x 10 cm x 10 cm square
• 10 cm x 10 cm x 10 cm cube (orange)

Use the four centimeter blocks to answer the following questions:
Let one orange rod = 1

If an orange rod = 1, then
one white cube =

If an orange rod = 1, then
one orange square =

If an orange rod = 1, then
one orange cube =

If one orange rod = 1, then what is the value of each of the following sets?

1 orange square, 2 orange rods, and 4 white cubes

8 orange squares and 3 white cubes

2 orange rods, and 8 white cubes

5 orange cube, 2 orange rods, and 4 white cubes

7 white cubes

1 orange square, 3 orange rods, and 2 white cubes

5 orange cubes, 4 orange squares, 6 white rods, and 3 white cubes

How many cubes, squares, and rods are needed to represent the following numerals?

1.4

1.5

2.5

12.6

Orange square with a white cube, orange rod, and orange square

Materials:

• 1 cm x 1 cm x 1cm cube (white)
• 1 cm x 1 cm x 10 cm rod
• 1 cm x 10 cm x 10 cm square
• 10 cm x 10 cm x 10 cm cube (orange)

Use the four centimeter blocks to answer the following questions
Let one orange square = 1

If an orange square = 1, then
one orange rod =

If an orange square = 1, then
one white cube =

If an orange square = 1, then
one orange cube =

If one orange square = 1, then what is the value of each of the following sets?

2 orange rods and 3 white cubes

1 orange rod, and 2 white cubes

3 white cubes

9 orange squares, 4 orange rods, and 5 white cubes

5 orange squares and 7 white cubes

2 orange cubes, 3 orange rods, and 8 white cubes

How many cubes, squares, and rods are needed to represent the following numerals?

4.01 3.6

5 7.13

1.05

10.12

Orange cube with a white cube, rod, and orange square

Materials:

• 1 cm x 1 cm x 1cm cube (white)
• 1 cm x 1 cm x 10 cm rod
• 1 cm x 10 cm x 10 cm square
• 10 cm x 10 cm x 10 cm cube (orange)

Use the four centimeter blocks to answer the following questions
Let one orange cube = 1

If an orange cube = 1, then
one square =

If an orange cube = 1, then
one orange rod =

If an orange cube = 1, then
one white cube =

8 orange squares, 3 orange rods, and 4 white cubes

3 orange cubes and 2 white cubes

3 orange cubes, 2 oranges squares, 6 orange rods, and 5 white cubes

8 orange squares, 5 orange rods, and 6 white cubes

6 orange squares and 7 white cubes

9 white cubes

4 orange cubes, 2 white rods, and 5 white cubes

How many cubes, squares, and rods are needed to represent the following numerals?

1.23

1.25

1.05

.001

.789

Review

Solve problems like the following:

If an orange rod = .1, then .......... = 1.

If an orange square = .1, then .......... = 1.

If a white cube = .01, then .......... = 1.

If a white cube = .001, then .......... = 1.

If an orange rod = .01, then .......... = 1.

Dr. Robert Sweetland's notes
[Home: homeofbob.com & thehob.net ]