Spinners and probability
Same Spinners Same Numbers
If two spinners are divided equally, numbered with three numbers, will either spinner be more likely than the other to have higher or lower numbers?
Purpose: To investigate equal probability, unequal probability, experimental probability, theoretical probability, tree diagrams, and tables with respect to probability.
Materials: sets of equal spinners with like numbers and unlike numbers (spinners).
Procedure:
Determine:
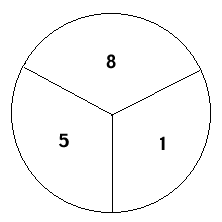
- If two players use the same spinner (each spins a 1, 2, 3 spinner).
- Determine who would be more likely to spin the smaller or larger number in ten spins?
- Experimental probability: Find a partner and play ten games.
- Record the outcomes on the chart below: (1, 2, 3), and which person has the high or low number or tie.
Is it more likely to spin smaller numbers?
Would this be a fair game?
Why or why not?
Game data:
| Game | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | Total |
| Spinner A 1 2 3 | |||||||||||
| High, Low, Tie | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | __ H __ L ___T |
| Spinner B 1 2 3 | |||||||||||
| High, Low, Tie | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | H-L-T | __ H __ L __ T |
Share the data with the class and complete the class data chart.
Class data for 12 teams:
| Spinner A 1 2 3 | Spinner B 1 2 3 | |||||
| Hight | Low | Tie | High | Low | Tie | |
| Team 1 | ||||||
| Team 2 | ||||||
| Team 3 | ||||||
| Team 4 | ||||||
| Team 5 | ||||||
| Team 6 | ||||||
| Team 7 | ||||||
| Team 8 | ||||||
| Team 9 | ||||||
| Team 10 | ||||||
| Team 11 | ||||||
| Team 12 | ||||||
| Total | ||||||
What is your experimental probability?
What is the class's experimental probability?
Theoretical probability: Use the tree diagram to determine all of the possible outcomes and record them in the chart below.
Possible outcomes tree:
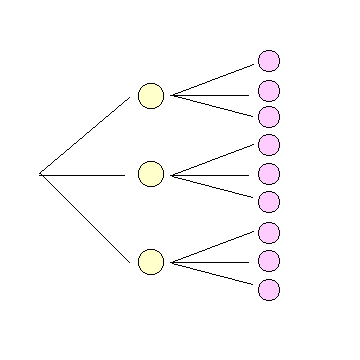 |
| Spinner A 1 | Spinner A 2 | Spinner A 3 | |
| Spinner B 1 | |||
| Spinner B 2 | |||
| Spinner B 3 |
What is the experimental probability?
Hints
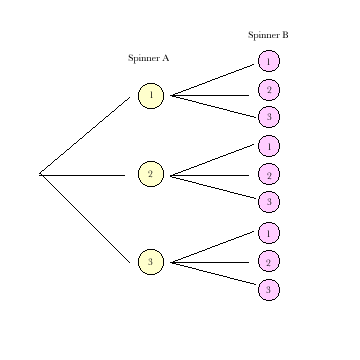 |
| Spinner A 1 | Spinner A 2 | Spinner A 3 | |
| Spinner B 1 | 1 1 tie | 1 2 spinner A | 1 3 spinner A |
| Spinner B 2 | 1 2 spinner B | 2 2 tie | 2 3 spinner A |
| Spinner B 3 | 3 1 spinner B | 2 3 spinner B | 3 3 tie |
Same Spinners Different Numbers
Purpose: To investigate equal probability, unequal probability, experimental probability, theoretical probability, tree diagrams, and tables with respect to probability.
Materials: sets of equal spinners with like numbers and unlike numbers (spinners).
Procedure
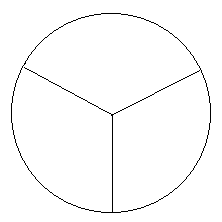
If two players use spinner divided into equal sections, but each has a different set of numbers on the spinner. Who would be more likely to spin the smaller or larger number in ten spins?
Experimental probability:
Find a partner and each spin one of the two different spinners (1, 5, 8 or 2, 4, 7) record the outcomes.
Is it more likely to spin smaller numbers?
Would this be a fair game?
Why or why not?
Game data:
| Game | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | Total |
| spinner 1 5 8 | |||||||||||
| High or Low | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | ____ H - ____ L |
| spinner 2 4 7 | |||||||||||
| High or Low | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | H - L | ____ H - ____ L |
Share the data with the class and complete the class data chart.
Class data for 12 teams:
| Spinner 1 5 8 | Spinner 2 4 7 | |||
| High | Low | High | Low | |
| Team 1 | ||||
| Team 2 | ||||
| Team 3 | ||||
| Team 4 | ||||
| Team 5 | ||||
| Team 6 | ||||
| Team 7 | ||||
| Team 8 | ||||
| Team 9 | ||||
| Team 10 | ||||
| Team 11 | ||||
| Team 12 | ||||
| Total | ||||
What is your experimental probability?
What is the class's experimental probability?
Theoretical probability: Use the tree diagram to determine all of the possible outcomes and record them in the chart below.
Possible outcomes tree:
 |
| Spinner 158 - 1 | Spinner 158 - 5 | Spinner 158 - 8 | |
| Spinner 247 - 2 | |||
| Spinner 247 - 4 | |||
| Spinner 247 - 7 |
What is the experimental probability?
Hints
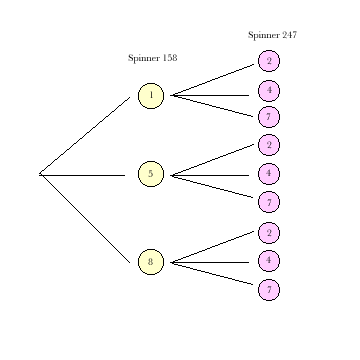 |
| Spinner 158 - 1 | Spinner 158 - 5 | Spinner 158 - 8 | |
| Spinner 247 - 2 | 2 1 spinner 247 | 2 5 spinner 158 | 2 8 spinner 158 |
| Spinner 247 - 4 | 4 1 spinner 247 | 4 5 spinner 158 | 4 8 spinner 158 |
| Spinner 247 - 7 | 7 1 spinner 247 | 7 5 spinner 247 | 7 8 spinner 158 |
How did you determine experimental probability?
How did you determine theoretical probability?
What is the difference between theoretical probability and experimental probability?
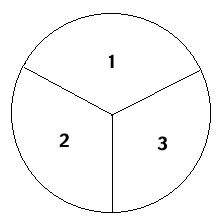
Spinners
Use a paper clip to make a spinner.
- Put the smaller end at the center of the circle, so the larger end is toward the edge of the circle.
- Put the point of your pen or pencil inside the paper clip and on the center of the circle.
- Flick the paper clip so that it spins around the point.
 |
 |
 |
 |